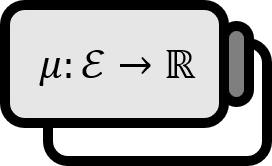📂測度論

空集合

定義 ¹

実数の区間の集合$\mathcal{I}$に対して、函数$l : \mathcal{I} \to [ 0 , \infty )$を$l( I ) := \sup{I} - \inf{I}$として定義して、長さ^lengthと言おう。任意の$\varepsilon > 0$に対して $$A \subset \bigcup_{n = 1}^{\infty} I_{n} \\ \sum_{n=1}^{\infty} l (I_{n}) < \varepsilon$$ を満たす区間の数列$\left\{ I_{n} \ | \ n \in \mathbb{N} \right\}$が存在する場合、$A \subset \mathbb{R}$を零集合^{null set}という。

Description

The set of all intervals is denoted as $\mathcal{I}$, and the set of all null sets is denoted as $\mathcal{N}$.

Be mindful that Null and Empty have a similar meaning but distinct nuances. The difference could be explained as Null being ‘practically’ nonexistent, whereas Empty feels ‘actually’ nonexistent. Upon reflection, in fields outside of mathematics, true ‘absence’ is difficult to find or prove. In some sense, this is why computer science prefers Null over Empty. For example, even if a variable is declared but not assigned any value, it is not considered an abstract ’nothingness’ but rather a ‘meaningless’ state. Since it’s meaningless, there’s no reason to preserve it using memory, and it can disappear at any time. Following this distinction, it seems quite appropriate to call it Null. Defining a null set in this way means a set is not technically empty but is ‘practically’ meaningless in terms of the concept of ’length’.

Theorem

• [1]: The empty set is a null set.
• [2]: A singleton set is a null set.
• [3]: A countable set is a null set.

Proof

[1]

Given $n \in \mathbb{N}$, let’s say $I_{n} : = \emptyset$, then $$\emptyset \subset \bigcup_{n = 1}^{\infty} I_{n} \\ \sum_{n=1}^{\infty} l (I_{n}) = 0 < \varepsilon$$

■

[2]

Consider a singleton set $\left\{ x \right\}$. Given $n \in \mathbb{N}$, saying $\displaystyle I_{n} : = \left( x - {{ \varepsilon } \over {2 \cdot 2^{n+1}}} , x + {{ \varepsilon } \over {2 \cdot 2^{n+1}}}\right)$ naturally leads to the following.

$$\left\{ x \right\} \subset \bigcup_{n = 1}^{\infty} I_{n} \\ \sum_{n=1}^{\infty} l (I_{n}) = {{\varepsilon} \over {2}} < \varepsilon$$

■

[3]

Strategy: This applies the idea of bijectively mapping natural numbers to rational numbers. On the other hand, as a counterexample to [3]’s inverse ‘a non-countable set is not a null set’, Cantor’s set shows it’s false.

---

According to Summary [1], as a singleton set is a null set, for a sequence of null sets $\left\{ N_{n} \right\}$, if $\displaystyle N : = \bigcup_{n=1}^{\infty} N_{n}$ is a null set, then it’s sufficient. Since $N_{1}$ is a null set,

$$\sum_{k=1}^{\infty} l (I_{(k,1)}) < {{\varepsilon} \over {2}} \\ N_{1} \subset \bigcup_{k=1}^{\infty} I_{(k,1)}$$ a $\left\{ I_{(k,1)} \ | \ k \in \mathbb{N} \right\}$ that satisfies this exists. Similarly, since $N_{n}$ is a null set, $$\sum_{k=1}^{\infty} l (I_{(k,n)}) < {{\varepsilon} \over {2^{n}}} \\ N_{n} \subset \bigcup_{k=1}^{\infty} I_{(k,n)}$$ a $\left\{ I_{(k,n)} \ | \ k \in \mathbb{N} \right\}$ that satisfies this exists. $$\begin{bmatrix} 1 & 2 & 6 & 7 & \cdots \\ 3 & 5 & 8 & \ddots & \\ 4 & 9 & \ddots & & \\ 10 & \ddots & & \\ \vdots & & \end{bmatrix} \mapsto \begin{bmatrix} (1,1) & (1,2) & (1,3) & (1,4) & \cdots \\ (2,1) & (2,2) & (2,3) & \ddots & \\ (4,1) & (4,2) & \ddots & & \\ (5,1) & \ddots & & \\ \vdots & & \end{bmatrix}$$

By defining a sequence of intervals with given indices $J_{j} : = I_{(k,n)}$ as above, $$N = \bigcup_{n=1}^{\infty} N_{n} \subset \bigcup_{n=1}^{\infty} \bigcup_{k=1}^{\infty} I_{(k,n)} = \bigcup_{j=1}^{\infty} J_{j}$$ and $$\sum_{j=1}^{\infty} l (J_{j}) = \sum_{n=1, k=1}^{\infty} l (I_{(k,n)}) = \sum_{n=1}^{\infty} \left( \sum_{k=1}^{\infty} l (I_{(k,n)}) \right) < \sum_{n=1}^{\infty} {{\varepsilon} \over { 2^{n} }} = \varepsilon$$ hence, $N$ is a null set.

■