두 벡터의 외적의 크기는 두 벡터가 만드는 평행사변형의 넓이와 같다
정리
두 벡터 $\mathbf{A}$, $\mathbf{B}$ 사이의 각도가 $\theta$일 때 두 벡터의 외적의 크기는 다음과 같다.
$$ \left| \mathbf{A}\times \mathbf{B}\right| =\left|\mathbf{A}\right|\left| \mathbf{B} \right|\sin \theta $$
그리고 이는 두 벡터가 만드는 평행사변형의 넓이와 같다.
증명

두 벡터 $\mathbf{A}=(A_{x},A_{y},A_{z})$, $\mathbf{B}=(B_{x},B_{y},B_{z})$가 위 그림과 같다고 하자. 그러면
- part 1. 평행사변형의 넓이
평행사변형의 넓이는 밑변과 높이의 곱이므로 아래와 같다.
$$ |\mathbf{A}| | \mathbf{B}|\sin\theta $$
- part 2. 외적의 크기
$$ \begin{align*} \left|\mathbf{A}\times \mathbf{B}\right|^{2} &= \left| (A_{y}B_{z}-A_{z}B_{y})\hat{\mathbf{x}}+(A_{z}B_{x}-A_{x}B_{z})\hat{\mathbf{y}}+(A_{x}B_{y}-A_{y}B_{x})\hat{\mathbf{z}} \right|^{2} \\ &=(A_{y}B_{z}-A_{z}B_{y})^{2}+(A_{z}B_{x}-A_{x}B_{z})^{2}+(A_{x}B_{y}-A_{y}B_{x})^{2} \\ &= A_{y}^{2}B_{z}^{2}-2A_{y}A_{z}B_{y}B_{z}+A_{z}^{2}B_{y}^{2} \\ &\quad+ A_{z}^{2}B_{x}^{2}-2A_{z}A_{x}B_{z}B_{x}+A_{x}^{2}B_{z}^{2} \\ &\quad+ A_{x}^{2}B_{y}^{2}-2A_{x}A_{y}B_{x}B_{y}+A_{y}^{2}B_{x}^{2} \\ &\quad \color{red}{+A_{x}^{2}B_{x}^{2}+A_{y}^{2}B_{y}^{2}+A_{z}^{2}B_{z}^{2}} \color{blue}{-A_{x}^{2}B_{x}^{2}-A_{y}^{2}B_{y}^{2}-A_{z}^{2}B_{z}^{2}} \\ &= A_{x}^{2}(B_{x}^{2}+B_{y}^{2}+B_{z}^{2})+ A_{y}^{2}(B_{x}^{2}+B_{y}^{2}+B_{z}^{2})+ A_{z}^{2}(B_{x}^{2}+B_{y}^{2}+B_{z}^{2}) \\ &\quad -(A_{x}^{2}B_{x}^{2}+A_{y}^{2}B_{y}^{2}+A_{z}^{2}B_{z}^{2})^{2} \\ &= (A_{x}^{2}+A_{y}^{2}+A_{z}^{2})(B_{x}^{2}+B_{y}^{2}+B_{z}^{2})-(A_{x}^{2}B_{x}^{2}+A_{y}^{2}B_{y}^{2}+A_{z}^{2}B_{z}^{2})^{2} \\ &= |\mathbf{A}|^{2}|\mathbf{B}|^{2}-|\mathbf{A}\cdot\mathbf{B}|^{2} \\ &= |\mathbf{A}|^{2}|\mathbf{B}|^{2}-|\mathbf{A}|^{2}|\mathbf{B}|^{2}\cos ^{2 }\theta \\ &=|\mathbf{A}|^{2}|\mathbf{B}|^{2}(1-\cos ^{2 }\theta) \\ &=|\mathbf{A}|^{2}|\mathbf{B}|^{2}\sin ^{2 } \theta \end{align*} $$
따라서
$$ \left|\mathbf{A} \times \mathbf{B} \right|=\left|\mathbf{A}\right|\left| \mathbf{B} \right|\sin \theta $$
■
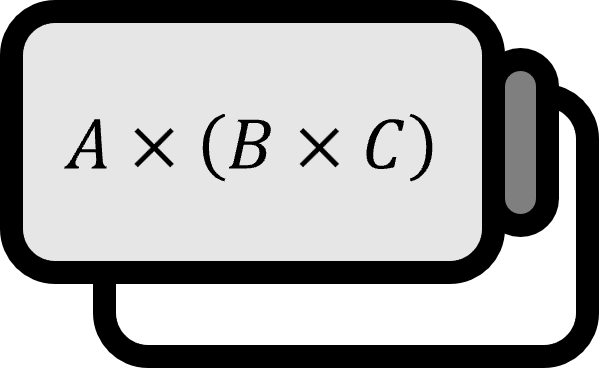
 저희들의 저서 「줄리아 프로그래밍」이 2024 세종도서 학술부문에 선정되었습니다!
저희들의 저서 「줄리아 프로그래밍」이 2024 세종도서 학술부문에 선정되었습니다!

