What is a Hessian Matrix?
Definition
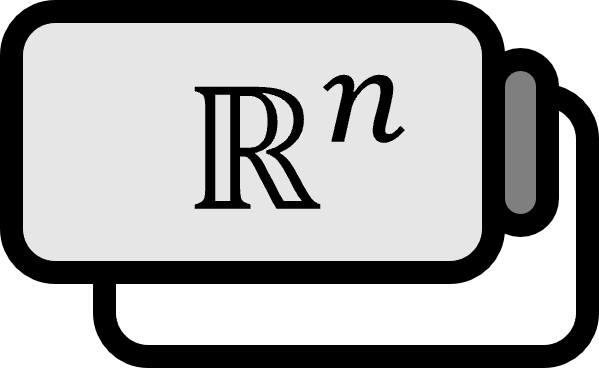
$D \subset \mathbb{R}^{n}$ is defined as the matrix $H \in \mathbb{R}^{n \times n}$ for a multivariate scalar function $f : D \to \mathbb{R}$ is called the Hessian matrix of $f$.
$$ H := \begin{bmatrix} {{\partial^2 f } \over {\partial x_{1}^2 }} & \cdots & {{\partial^2 f } \over { \partial x_{1} \partial x_{n} }} \\ \vdots & \ddots & \vdots \\ {{\partial^2 f } \over {\partial x_{n} \partial x_{1} }} & \cdots & {{\partial^2 f_{m} } \over {\partial x_{n}^2 }} \end{bmatrix} $$
Description
For the Hessian of $f$, the following notations are used.
$$ H,\quad H(f),\quad H_{f},\quad \mathbf{H},\quad \nabla^{2}f $$
Note that $\nabla^{2}$ is often used as a notation for the Laplacian.
If the Jacobian matrix corresponds to the high-dimensional derivative of a function, then the Hessian matrix can be seen as the high-dimensional second derivative. It might not appear as frequently as the Jacobian matrix, but it does appear occasionally in unexpected places like mathematical statistics. Also, note that the Hessian matrix is defined only for scalar functions.