📂Data Science

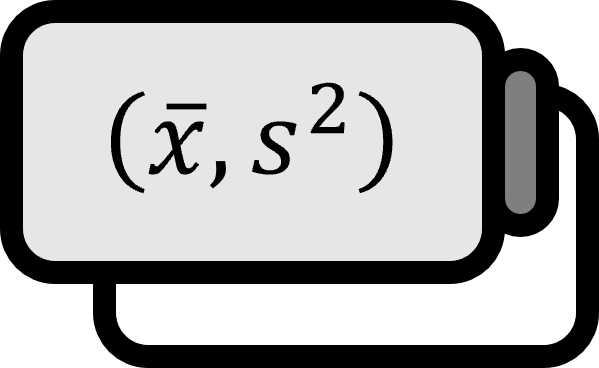
Definition of Variance in Basic Statistics

Definition ¹

Let’s assume we are given $n$ quantitative data.

1. The difference $\left( \overline{x} - x_{i} \right)$ between the sample mean $\overline{x}$ and the data is called the deviation.
2. The value $s^{2}$, which is the sum of the squares of deviations divided by $n-1$, is known as the variance of a sample. $$s^{2} := {{ \sum \left( x_{i} - \overline{x} \right)^{2} } \over { n-1 }}$$ Taking the square root of the sample variance $s = \sqrt{s^{2}}$ is referred to as the standard deviation.

Description

Dispersion refers to how spread out the data is, and it is also known as variability or spread. Variance is a measure of this dispersion and is considered the second most important statistic after the mean.

See Also

When first encountering statistics, one might wonder why calculations involve squaring and why dividing by $n-1$ instead of $n$; these points can seem troublesome. As one majors in statistics and advances in their studies, they will encounter mathematical theories (which are by no means easy) that provide answers to these questions. If you are a freshman, it’s okay to just accept it for now.

• Reasons for dividing the sample variance by $n-1$
• Mathematical properties of descriptive statistics: The mean has the property of minimizing variance.
• Mathematical definition of variance in statistics