📂Linear Algebra

The Eigenvalues of the Null Matrix are Only Zero

Theorem¹

English Translation:

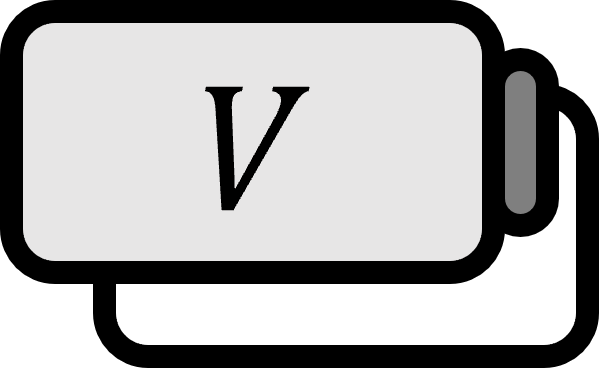
Let’s consider $V$ as a finite-dimensional vector space, and $T : V \to V$ as a nilpotent linear transformation. Then, the eigenvalues of $T$ are exclusively $0$.

Japanese Translation:

$V$を有限次元のベクトル空間、$T : V \to V$を冪零の線形変換としよう。すると、$T$の固有値は$0$だけだ。