삼각함수의 정의
개요
삼각함수는 직각삼각형의 밑각에 삼각비를 대응시킨 함수다.
정의

삼각함수 사인, 코사인 $\sin, \cos : \mathbb{R} \to \mathbb{R}$ 는 다음과 같이 정의된다.
$$ \sin \theta := {{ y } \over { \sqrt{x^{2} + y^{2}} }} \\ \cos \theta := {{ x } \over { \sqrt{x^{2} + y^{2}} }} $$
이에 따라 시컨트, 코시컨트, 탄젠트, 코탄젠트를 다음과 같이 정의한다.
$$ \begin{align*} \tan \theta &:= {{ \sin \theta } \over { \cos \theta }} \qquad, \cos \theta \ne 0 \\ \cot \theta &:= {{ \cos \theta } \over { \sin \theta }} \qquad, \sin \theta \ne 0 \\ \sec \theta &:= {{ 1 } \over { \cos \theta }} \qquad, \cos \theta \ne 0 \\ \csc \theta &:= {{ 1 } \over { \sin \theta }} \qquad, \sin \theta \ne 0 \end{align*} $$
복소함수로의 확장 1
삼각함수 사인, 코사인 $\sin, \cos : \mathbb{C} \to \mathbb{C}$ 는 다음과 같이 정의된다.
$$ \sin z := {{ 1 } \over { i2 }} \left( e^{iz} - e^{-iz} \right) \\ \cos z := {{ 1 } \over { 2 }} \left( e^{iz} + e^{-iz} \right) $$
기초 성질
- [1] 삼각함수는 실수축에서 $2 \pi$-주기함수다.
- [2] 사인함수는 기함수고, 코사인함수는 우함수다.
같이보기
Osborne (1999). Complex variables and their applications: p28. ↩︎
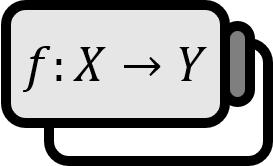
 저희들의 저서 「줄리아 프로그래밍」이 2024 세종도서 학술부문에 선정되었습니다!
저희들의 저서 「줄리아 프로그래밍」이 2024 세종도서 학술부문에 선정되었습니다!

