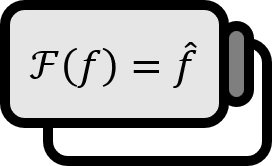📂フーリエ解析

コンボリューションのサポート

定理

実数の二つの集合 $A, B$に対して、$A + B$を次のように定義しよう。

$$A + B := \left\{ a + b : \forall a \in A, \forall b \in \supp B \right\}$$

二つの関数 $f, g$に対して以下が成立する。

$$\supp f \ast g \subset \supp f + \supp g$$

ここで、$\supp$は関数のサポートであり、$\ast$はコンボリューションである。

証明¹

$x \notin \supp f + \supp g$と仮定しよう。するとどんな$y$を選んでも$f(y)g(x-y)=0$となる。

• ケース 1 $y \in \supp f$

  この場合、$x - y \notin \supp g$となる。もし$x - y \in \supp g$と仮定するならば、

  $$\supp f + \supp g \ni (x - y) + y = x \notin \supp f + \supp g$$

  これは矛盾を導く。従って $x - y \notin \supp g$であり、$g(x-y) = 0$である。

• ケース 2 $y \notin \supp f$

  この場合、$f(y) = 0$である。

よって、$x \notin \supp f + \supp g$であれば、$\displaystyle \int f(y)g(x - y) dy = f \ast g(x) = 0$となり、$x \notin \supp f \ast g$となる。従って、

$$\supp f \ast g \subset \supp f + \supp g$$

■