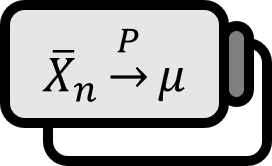📂数理統計学

共分散行列

定義¹

$p$次元ランダムベクトル$\mathbf{X} = \left( X_{1}, \cdots , X_{p} \right)$に対して、次のように定義された$\operatorname{Cov} (\mathbf{X})$を共分散行列^{covariance matrix}という。

$$ \left( \operatorname{Cov} \left( \mathbf{X} \right) \right)_{ij} := \operatorname{Cov} \left( X_{i} , X_{j} \right) $$

---

• $\operatorname{Cov}$は共分散である。

説明

定義をもっと簡単に説明すると次の通り。

$$ \operatorname{Cov} \left( \mathbf{X} \right) := \begin{pmatrix} \Var \left( X_{1} \right) & \operatorname{Cov} \left( X_{1} , X_{2} \right) & \cdots & \operatorname{Cov} \left( X_{1} , X_{p} \right) \\ \operatorname{Cov} \left( X_{2} , X_{1} \right) & \Var \left( X_{2} \right) & \cdots & \operatorname{Cov} \left( X_{2} , X_{p} \right) \\ \vdots & \vdots & \ddots & \vdots \\ \operatorname{Cov} \left( X_{p} , X_{1} \right) & \operatorname{Cov} \left( X_{p} , X_{2} \right) & \cdots & \Var \left( X_{p} \right) \end{pmatrix} $$

すべての共分散行列は半正定値行列である。つまり、すべてのベクトル$\mathbf{x} \in \mathbb{R}^{p}$に対して次が成り立つ。

$$ 0 \le \textbf{x}^{T} \operatorname{Cov} \left( \mathbf{X} \right) \textbf{x} $$

定理

• [1]: $\mathbf{\mu} \in \mathbb{R}^{p}$が$\mathbf{\mu} := \left( EX_{1} , \cdots , EX_{p} \right)$のように与えられているとすると $$ \operatorname{Cov} (\mathbf{X}) = E \left[ \mathbf{X} \mathbf{X}^{T} \right] - \mathbf{\mu} \mathbf{\mu}^{T} $$

• [2]: 定数の行列$A \in \mathbb{R}^{k \times p}$が$(A)_{ij} := a_{ij}$のように与えられているとすると $$ \operatorname{Cov} ( A \mathbf{X}) = A \operatorname{Cov} \left( \mathbf{X} \right) A^{T} $$

• $$ \Cov \left( \mathbf{X} \right) = [\Cov \left( \mathbf{X} \right)]^{T} $$

---

• $A^{T}$は$A$の転置行列である。

証明

[1]

$$ \begin{align*} \operatorname{Cov} \left( \mathbf{X} \right) =& E \left[ \left( \mathbf{X} - \mathbf{\mu} \right) \left( \mathbf{X} - \mathbf{\mu} \right)^{T} \right] \\ =& E \left[ \mathbf{X} \mathbf{X}^{T} - \mathbf{\mu} \mathbf{X}^{T} - \mathbf{X} \mathbf{\mu}^{T} + \mathbf{\mu} \mathbf{\mu}^{T} \right] \\ =& E \left[ \mathbf{X} \mathbf{X}^{T} \right] - \mathbf{\mu} E \left[ \mathbf{X}^{T} \right] - E \left[ \mathbf{X} \right] \mathbf{\mu}^{T} + E \left[ \mathbf{\mu} \mathbf{\mu}^{T} \right] \\ =& E \left[ \mathbf{X} \mathbf{X}^{T} \right] - \mathbf{\mu} \mathbf{\mu}^{T} \end{align*} $$

■

[2] ²

$$ \begin{align*} \operatorname{Cov} \left( A \mathbf{X} \right) =& E \left[ \left( A\mathbf{X} - A\mathbf{\mu} \right) \left( A\mathbf{X} - A\mathbf{\mu} \right)^{T} \right] \\ =& E \left[ A\left(\mathbf{X} -\mathbf{\mu} \right) \left( \mathbf{X} - \mathbf{\mu} \right)^{T} A^{T} \right] \\ =& A E \left[ \left(\mathbf{X} -\mathbf{\mu} \right) \left( \mathbf{X} - \mathbf{\mu} \right)^{T}\right] A^{T} \\ =& A \operatorname{Cov}\left( \mathbf{X} \right) A^{T} \end{align*} $$

■

3

共分散が$\Cov(X, Y) = \Cov(Y, X)$を満たすため成立する。

■