📂Linear Algebra

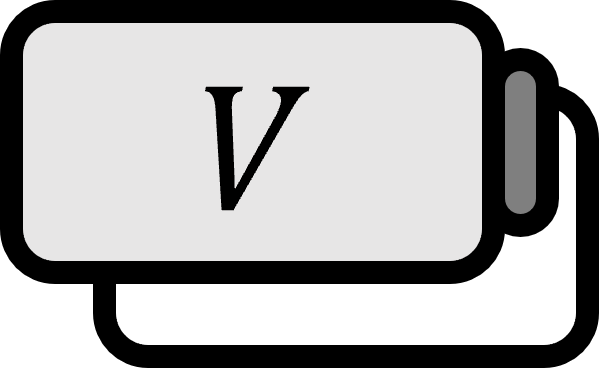
Reflexive of Vector Spaces

Definition ¹

If $X$ is a vector space and $X^{\ast \ast}$ is its bidual, then $X$ is said to be reflexive if $X^{\ast \ast} \approx X$.

Explanation

Generally, the size of a vector space increases with each dual taken. However, reflexivity essentially means that the dual space does not continue to grow. Examples of reflexive spaces include:

• For $1 < p < \infty$, then ${\ell^{p}}^{\ast \ast} \approx \ell^{p}$
• If $\dim X = n$, then $X^{\ast \ast} \approx X$
• If $H$ is a Hilbert space, then $H^{\ast \ast} \approx H$

An introduction to the proof concerning $\ell^{p}$ spaces.

Proof

Strategy: Define the function $\operatorname{sign} : \mathbb{C} \to \mathbb{R}$ specifically for this proof. $$ \operatorname{sign} ( \lambda ) := \begin{cases} \displaystyle {{| \lambda | } \over { \lambda }} &, \lambda \ne 0 \\ 1 &, \lambda = 0 \end{cases} $$ By definition, $\lambda \operatorname{sign} (\lambda) = | \lambda |$. Note that this slightly differs from the widely used definition of the sign of a complex number $\operatorname{sign}$.

---

First, we will prove that for $\displaystyle {{1} \over {p}} + {{1} \over {q}} = 1$, ${\ell^{p}}^{ \ast } \approx \ell^{q}$ holds. Define the map $\phi : {\ell^{p}}^{ \ast } \to \ell^{q}$ for $f \in {\ell^{p}}^{ \ast }$ as $\phi (f) = \left( f(e_{1}) , f(e_{2}) , \dots \right)$, where $e_{j}$ represents the unit vector $e_{j}:=(\dots ,0, 1 , 0 , \dots )$ that is $1$ in the $j$st component and $0$ in others.

Now, define $\displaystyle y_{j} : = {{ \operatorname{sign} \left( f^q (e_{j} ) \right) f^{q-1} (e_{j} ) } \over { \left( \sum_{j=1}^{n} \left| f (e_{j} ) \right|^{q} \right)^{{{1} \over {p}}} }} \in \mathbb{C}$ containing $\displaystyle y_{j} : = {{ \operatorname{sign} \left( f^q (e_{j} ) \right) f^{q-1} (e_{j} ) } \over { \left( \sum_{j=1}^{n} \left| f (e_{j} ) \right|^{q} \right)^{{{1} \over {p}}} }} \in \mathbb{C}$. Since for all $\lambda \in \mathbb{C}$, $|\lambda| = 1$,

$$ \| y_{1} e_{1} + \dots + y_{n} e_{n} \|_{p}^{p} = \sum_{j=1}^{n} |y_{j}|^{p} = \sum_{j=1}^{n} {{ 1 \cdot \left| f (e_{j} ) \right|^{(q-1)p} } \over { \left( \sum_{j=1}^{n} \left| f (e_{j} ) \right|^{q} \right) }} $$

it follows that $(q-1)p = q$; therefore,

$$ \| y_{1} e_{1} + \dots + y_{n} e_{n} \|_{p}^{p} = 1 $$

Since $f \in \ell_{p}^{ \ast }$ is linear,

$$ \begin{align*} & f( y_{1} e_{1} + \dots + y_{n} e_{n} ) \\ =& y_{1} f( e_{1} ) + \dots + y_{n} f(e_{n}) \\ =& {{ 1 } \over { \left( \sum_{j=1}^{n} \left| f (e_{j} ) \right|^{q} \right)^{{{1} \over {p}}} }} \left( \operatorname{sign} \left( f^q (e_{1} ) \right) f^{q-1} (e_{1} ) f(e_{1}) + \dots +\operatorname{sign} \left( f^q (e_{n} ) \right) f^{q-1} (e_{n} ) f(e_{n}) \right) \\ =& {{ 1 } \over { \left( \sum_{j=1}^{n} \left| f (e_{j} ) \right|^{q} \right)^{{{1} \over {p}}} }} \left( \operatorname{sign} \left( f^q (e_{1} ) \right) f^{q} (e_{1} )+ \dots +\operatorname{sign} \left( f^q (e_{n} ) \right) f^{q} (e_{n} ) \right) \end{align*} $$

$$\lambda \operatorname{sign} (\lambda) = | \lambda |$$

Following the property of $\operatorname{sign}$,

$$ \begin{align*} f( y_{1} e_{1} + \dots + y_{n} e_{n} ) =& {{ 1 } \over { \left( \sum_{j=1}^{n} \left| f (e_{j} ) \right|^{q} \right)^{{{1} \over {p}}} }} \left( \left| f (e_{1}) \right|^{q} + \dots + \left| f (e_{n}) \right|^{q} \right) \\ =& \left( \sum_{j=1}^{n} \left| f(e_{j} ) \right|^{q} \right)^{1- {{1} \over {p}} } \\ =& \left( \sum_{j=1}^{n} \left| f(e_{j} ) \right|^{q} \right)^{ {{1} \over {q}} } \end{align*} $$

• Part 1. $\displaystyle \left| \left( \sum_{j=1}^{\infty} \left| f(e_{j} ) \right|^{q} \right)^{ {{1} \over {q}} } \right| \le \| f \|$

  Since $f$ is bounded, for all $n \in \mathbb{N}$,

  $$ \left| \left( \sum_{j=1}^{n} \left| f(e_{j} ) \right|^{q} \right)^{ {{1} \over {q}} } \right| \le \| f \| \| y_{1} e_{1} + \dots + y_{n} e_ {n} \|_{p} $$

  it follows from $\displaystyle \| y_{1} e_{1} + \dots + y_{n} e_{n} \|_{p}^{p} = 1$ that

  $$ \left| \left( \sum_{j=1}^{\infty} \left| f(e_{j} ) \right|^{q} \right)^{ {{1} \over {q}} } \right| \le \| f \| $$

• Part 2. $\phi$ is a function.

  From Part 1., since $\displaystyle \left| \phi (f) \right| = \left| \left( \sum_{j=1}^{\infty} \left| f(e_{j} ) \right|^{q} \right)^{ {{1} \over {q}} } \right| \le \| f \| < \infty$, then $\phi ( f ) \in \ell^{q}$

• Part 3. $\phi$ is linear.

  For $f , g \in {\ell^{p}}^{ \ast }$ and $\lambda \in \mathbb{C}$,

  $$ \begin{align*} \phi ( \lambda f + g ) =& \left( ( \lambda f + g ) e_{1} , \dots \right) \\ =& \left( \lambda f (e_{1}) + g (e_{1}) , \dots \right) \\ =&\left( \lambda f (e_{1}) , \dots \right) + \left( g (e_{1}) , \dots \right) \\ =& \lambda \phi (f) + \phi (g) \end{align*} $$

• Part 4. $\phi$ is injective.

  If for $f , g \in {\ell^{p}}^{ \ast }$, we say $\phi (f) = \phi (g)$, then for all $j \in \mathbb{N}$, $f(e_{j} ) = g( e_{j} )$ must hold. Let $( x_{j} ) \in \ell^{q}$,

  $$ f \left( ( x_{j} ) \right) = f \left( \lim_{n \to \infty} \left( x_{1} e_{1} + \dots + x_{n} e_{n} \right) \right) $$

  Since $\phi$ is linear, it is continuous,

  $$ \begin{align*} f \left( \lim_{n \to \infty} \left( x_{1} e_{1} + \dots + x_{n} e_{n} \right) \right) =& \lim_{n \to \infty} f \left( x_{1} e_{1} + \dots + x_{n} e_{n} \right) \\ =& \lim_{n \to \infty} \left( x_{1} f (e_{1} ) + \dots + x_{n} f(e_{n}) \right) \\ =& \lim_{n \to \infty} \left( x_{1} g (e_{1} ) + \dots + x_{n} g (e_{n}) \right) \\ =& \lim_{n \to \infty} g \left( x_{1} e_{1} + \dots + x_{n} e_{n} \right) \\ =& g \left( \lim_{n \to \infty} \left( x_{1} e_{1} + \dots + x_{n} e_{n} \right) \right) \\ =& g \left( ( x_{j} ) \right) \end{align*} $$

  summarizing,

  $$ \phi (f) = \phi (g) \implies f = g $$

• Part 5. $\phi$ is surjective.

  To show that for any $( \lambda_{j} ) \in \ell^{q}$, there exists $f_{0} \in {\ell^{p}}^{ \ast }$ satisfying $\phi ( f_{0} ) = ( \lambda_{j} )$. Define function $f_{0} : \ell^{p} \to \mathbb{C}$ as $\displaystyle f_{0} \left( (x_{j} ) \right) : = \sum_{j=1}^{\infty} x_{j} \lambda_{j}$. Thus, for $( x_{j} ) , ( y_{j} ) \in \ell^{p}$,

  $$ \begin{align*} f_{0} \left( \lambda ( x_{j} ) + ( y_{j} ) \right) =& f_{0} \left( ( \lambda x_{j} + y_{j} ) \right) \\ =& \sum_{j=1}^{\infty} ( \lambda x_{j} + y_{j} ) \lambda_{j} \\ =& \lambda \sum_{j=1}^{\infty} x_{j} \lambda_{j} + \sum_{j=1}^{\infty} y_{j} \lambda_{j} \\ =& \lambda f \left( (x_{j} ) \right) + f \left( (y_{j} ) \right) \end{align*} $$

  leading to $f_{0}$ being linear. Furthermore, by the Hölder inequality,

  $$ \| f_{0} \| = \sup_{ \| (x_{j}) \|_{p } = 1 } \left| \sum_{j=1}^{\infty} x_{j} \lambda_{j} \right| \le \sup_{ \| (x_{j}) \|_{p } = 1 } \left( \sum_{j=1}^{\infty} | x_{j} |^{p} \right)^{{1} \over {p}} \left( \sum_{j=1}^{\infty} | \lambda_{j} |^{q} \right)^{{1} \over {q}} < \infty $$

  thus, $f_{0}$ is bounded, concluding $f_{0} \in {\ell^{p}}^{ \ast }$. This $f_{0}$ also

  $$ \phi (f_{0} ) = \left( f_{0} (e_{1}) , f_{0} (e_{2}) , \dots \right) = \left( \sum_{j=1}^{\infty} e_{1} \lambda_{j} , \sum_{j=1}^{\infty} e_{2} \lambda_{j} , \dots \right) = (\lambda_{1} , \lambda_{2} , \dots ) = (\lambda_{j}) $$

  satisfies the requirement.

• Part 6. $\phi$ preserves norms.

  It is sufficient to show $\| \phi (f) \|_{q} = \| f \|$.

  $$ \begin{align*} \| f \| =& \sup_{ \| (x_{j}) \|_{p } = 1 } \left| f((x_{j} )) \right| \\ =& \sup_{ \| (x_{j}) \|_{p } = 1 } \left| \sum_{j=1}^{\infty} (x_{j} ) f(e_{j} ) \right| \\ \le & \sup_{ \| (x_{j}) \|_{p } = 1 } \sum_{j=1}^{\infty} | (x_{j} ) | | f(e_{j} ) | \le \sup_{ \| (x_{j}) \|_{p } = 1 } \left( \sum_{j=1}^{\infty} | x_{j} |^{p} \right)^{{1} \over {p}} \left( \sum_{j=1}^{\infty} | f ( e_{j} ) |^{q} \right)^{{1} \over {q}} \\ =& \left( \sum_{j=1}^{\infty} | f ( e_{j} ) |^{q} \right)^{{1} \over {q}} \\ =& \| \phi (f) \|_{q} \end{align*} $$

  However, from Part 1., since $\displaystyle \left| \left( \sum_{j=1}^{\infty} \left| f(e_{j} ) \right|^{q} \right)^{ {{1} \over {q}} } \right| \le \| f \|$,

  $$ \| f \| \le \| \phi (f) \|_{q} \le \| f \| $$

  summarizing,

  $$ \| \phi (f) \|_{q} = \| f \| $$

  Summarizing from Part 2. to Part 6., we see that $\phi$ is an isometry, thus proving that for $\displaystyle {{1} \over {p}} + {{1} \over {q}} = 1$, ${\ell^{p}}^{ \ast } \approx \ell^{q}$ holds.

• Part 7.
  If ${\ell^{p}}^{ \ast } \approx \ell^{q}$, then ${\ell^{p}}^{\ast \ast} \approx {\ell^{q}}^{ \ast }$ follows. Since isometry is an equivalence relation, and by the transitivity of equivalence relations,

  $$ \ell_{p}^{\ast \ast} \approx \ell_{p} $$

■