Euclidean Space

Definition

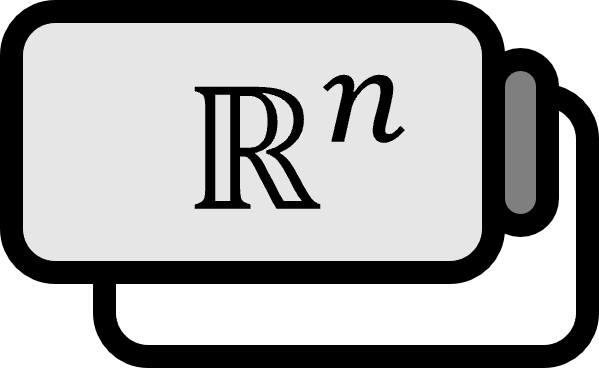
For a natural number $n \in \mathbb{N}$, the Cartesian product $\mathbb{R}$ of the set of real numbers is called the Euclidean space.

$$ \mathbb{R}^{n} = \mathbb{R} \times \cdots \times \mathbb{R} $$

$\mathbb{R}^{1}$ is referred to as real space or number line.
$\mathbb{R}^{2}$ is called a plane.
$\mathbb{R}^{3}$ is called a $3$-dimensional space.

Here, $\mathbb{N} := \left\{ 1, 2, 3, \cdots \right\}$ means the set that includes all natural numbers. $\mathbb{R}$ represents the set that includes all real numbers.

Explanation

The Euclidean space, named after Euclid, the author of Elements, is a space that describes not only our living $3$-dimensional space, including planes and number lines but also spaces of higher dimensions.

Being closely related to our lives, it is often assumed as the entire space in many theories. However, the Euclidean space alone cannot explain all the deep and profound theories of science and engineering and sometimes becomes a toy for pseudo-mathematicians and pseudo-scientists prolific on YouTube.

The extension to higher dimensions is necessary not necessarily for fancy purposes like being because of spacetime $\mathbb{R}^{1+3}$ or string theory where $\mathbb{R}^{11}$. For example, there are applications in statistics, and even if the space itself is $3$-dimensional, introducing speed and acceleration may require $9$ dimensions. If the reader is primarily interested in engineering or practical physics only, they might never need to venture beyond classical Euclidean space. Dreaming romantic notions from pop-science books without formulas and proofs can’t be helped. However, if one is interested in the worlds beyond math and physics, it’s pivotal to get familiar with Euclidean spaces sooner rather than later.