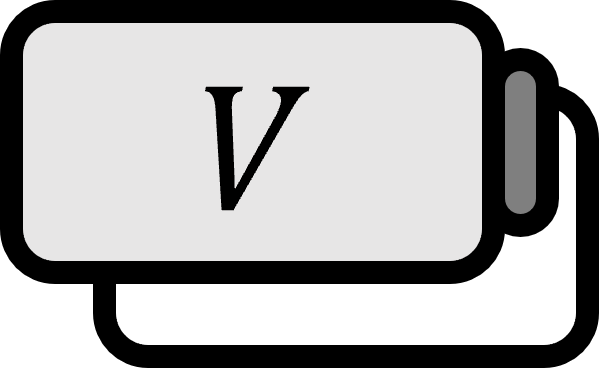
Sum of Subspaces in a Vector Space
Definition1
Let’s refer to $W_{1}, W_{2}$ as a subspace of the vector space $V$. The sum of $W_{1}$ and $W_{2}$ is denoted as $W_{1} + W_{2}$ and defined as follows.
$$ W_{1} + W_{2} := \left\{ x + y : x\in W_{1}, y \in W_{2} \right\} $$
Generalization2
Let $W_{1}, W_{2}, \dots, W_{k}$ be a subspace of the vector space $V$. The sum of these subspaces is denoted as $W_{1} + \cdots + W_{k}$ and defined as follows.
$$ W_{1} + \cdots + W_{k} = \sum\limits_{i=1}^{k}W_{i} := \left\{ v_{1} + \cdots + v_{k} : v_{i} \in W_{i} \text{ for } 1 \le i \le k \right\} $$
Explanation
It doesn’t have to be a subspace; a subset can be defined without any issues.
As the definition suggests, it is not necessarily required to be a vector space; as long as the addition of elements is well-defined, it works. Therefore, $W_{1}$ and $W_{2}$ could be subgroups of a group and the definition would still apply. Conversely, without the addition of elements, it cannot be defined.