📂Linear Algebra

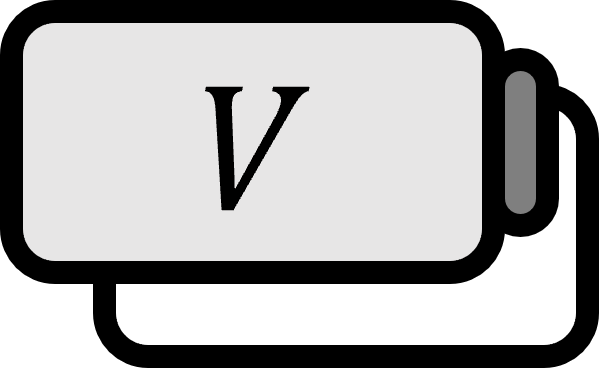
Linear Transformations on the Quotient Space

Definition ¹

Let $V$ be a vector space and $T : V \to V$ be a linear transformation. Let $W \le V$ be a $T$-invariant subspace. The linear transformation on quotient space $\overline{T}$ is defined as follows:

$$ \begin{align*} \overline{T} : V/W &\to V/W \\ v + W &\mapsto T(v) + W \end{align*} $$

Here, $V/W$ is the quotient space.

Theorem

(a) $\overline{T}$ is well-defined.

(b) $\overline{T}$ is indeed a linear transformation.

(c) For the mapping to the quotient space $\eta : V \to V/W$, $\eta T = \overline{T} \eta$ holds. That is, the following diagram is commutative:

$$ \begin{CD} V @>T>> V \\\ @VV \eta V @VV \eta V \\\ V/W @> \overline{T} >> V/W \end{CD} $$

Proof

(a)

It suffices to show that when $v_{1} + W = v_{2} + W$, then $\overline{T}(v_{1} + W) = \overline{T}(v_{2} + W)$ holds. Assume $v_{1} + W = v_{2} + W$. By the properties of cosets, this is equivalent to $v_{1} - v_{2} \in W$. Therefore, since $W$ is $T$-invariant and $T$ is a linear transformation,

$$ T(v_{1}) - T(v_{2}) = T(v_{1} - v_{2}) \in W $$

Therefore, since $T(v_{1}) - T(v_{2}) \in W \iff T(v_{1}) + W = T(v_{2}) + W$,

$$ v_{1} + W = v_{2} + W \implies \overline{T}(v_{1} + W) = \overline{T}(v_{2} + W) $$

■

(b)

Addition and scalar multiplication of cosets

$$ (v_{1} + W) + (v_{2} + W) = (v_{1} + v_{2}) + W,\quad \forall v_{1}, v_{2} \in V $$

$$ a(v + W) = av + W\quad \forall v \in V \text{ and } a \in F $$

Since $T$ is a linear transformation,

$$ \begin{align*} \overline{T}\left( (av_{1} + v_{2}) + W \right) &= T(av_{1} + v_{2}) + W \\ &= \left( aT(v_{1}) + T(v_{2}) \right) + W \\ &= \left( aT(v_{1}) + W \right) + \left( T(v_{2}) + W \right) \\ &= a\left( T(v_{1}) + W \right) + \overline{T}(v_{2} + W) \\ &= a\overline{T}(v_{1} + W) + \overline{T}(v_{2} + W) \\ \end{align*} $$

■

(c)

It can easily be shown by definition.

$$ \begin{align*} \eta\left( T(v) \right) &= T(v) + W \\ &= \overline{T}(v + W) \\ &= \overline{T}\left( \eta (v) \right) \end{align*} $$

■